Angol nyelvű matematika
|
9. évfolyam, 3. kiadás (2024. 02. 14.) Mozaik Kiadó
méret: 170x240 mm
terjedelem: 276 oldal
tanterv: NAT 2012
6 480 Ft
Kosárba  |
| A kiadvány az MS-2309 Sokszínű matematika 9. c. kötet angol nyelvű változata. This book is the English version of the former Hungarian market leader textbook titled Sokszínű matematika 9. |
Kapcsolódó kiadványok
MS-2309U2 980 Ft
MS-2377U4 280 Ft
MS-23234 480 Ft
MS-23284 280 Ft
MS-63116 480 Ft
MS-63126 480 Ft
MS-63106 480 Ft
| A kiadvány digitális változata a könyvben levő kóddal ingyenesen elérhető * A kiadvány hátsó borítójának belső oldalán található egyedi kóddal a kiadvány digitálisan is elérhető. Az aktivált kódokkal 1 éves DÍJMENTES hozzáférést ajándékozunk a kiadvány digitális változatához. Az aktiválás a www.mozaweb.hu/aktivalas oldalon, a Fiókom/Új kód aktiválása menüpontban érhető el. |
Mintaoldalak
Tartalomjegyzék
| Combinatorics, sets | 10 |
| 1. The meaning of expressions in the mathematical language | 10 |
| 2. Let us count up! | 15 |
| 3. Sets | 21 |
| 4. Set operations | 26 |
| 5. Order of sets, inclusion-exclusion principle | 32 |
| 6. Number lines, intervals | 36 |
| 7. Graphs | 38 |
| Algebra and arithmetics | 44 |
| 1. Usage of letters in mathematics | 44 |
| 2. Exponentiation | 48 |
| 3. Exponentiation to integer index | 52 |
| 4. Standard index form of numbers | 55 |
| 5. Integral expressions (polynomials) | 58 |
| 6. Special algebraic products | 60 |
| 7. Methods of factorisation | 66 |
| 8. Operations with algebraic fractions | 68 |
| 9. Divisibility | 74 |
| 10. Greatest common divisor (GCD), least common multiple (LCM) | 80 |
| 11. Number systems | 83 |
| Functions | 88 |
| 1. The Cartesian coordinate system, point sets | 88 |
| 2. Linear functions | 92 |
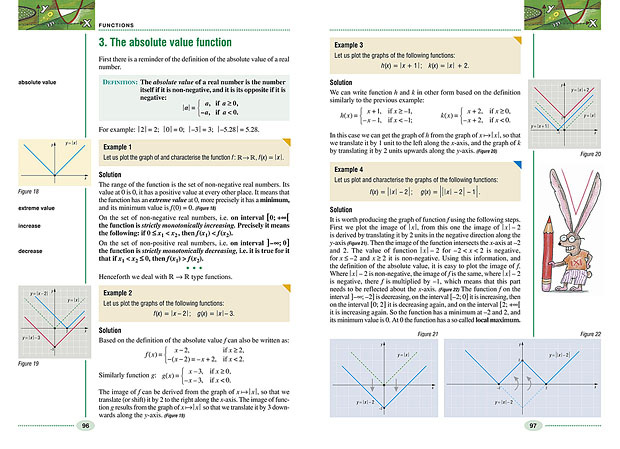
| 3. The absolute value function | 96 |
| 4. The quadratic function | 102 |
| 5. The square root function | 106 |
| 6. Linear fractional functions | 110 |
| 7. The integral part, the fractional part and the algebraic sign function (higher level courseware) | 116 |
| 8. More examples of functions (higher level courseware) | 120 |
| 9. Systematization of function transformations | 124 |
| Triangles, quadrilaterals, polygons | 128 |
| 1. Points, straight lines, planes and their mutual position . | 128 |
| 2. A few basic geometric concepts (reminder) | 129 |
| 3. About the triangles (reminder) | 133 |
| 4. The relation between the sides and the angles of the triangle | 135 |
| 5. The relation between the sides of a right-angled triangle | 136 |
| 6. About the quadrilaterals (reminder) | 139 |
| 7. About the polygons | 143 |
| 8. Special point sets . | 145 |
| 9. The inscribed circle of a triangle | 149 |
| 10. The circumscribed circle of a triangle | 151 |
| 11. Thales’ theorem and some of its applications . | 153 |
| 12. Circumscribed quadrilaterals, circumscribed polygons (higher level courseware) | 157 |
| Equations, inequalities, simultaneous equations | 160 |
| 1. The concept of equation, identity | 160 |
| 2. Solving equations graphically | 164 |
| 3. Solving equations with examining the domain and the range | 166 |
| 4. Solving equations with factorisation | 169 |
| 5. Solving equations with elimination, with the “balance method” | 173 |
| 6. Inequalities | 177 |
| 7. Equations and inequalities containing absolute value | 182 |
| 8. Parametric equations (higher level courseware) | 188 |
| 9. Solving problems with equations I | 191 |
| 10. Solving problems with equations II | 195 |
| 11. First-order simultaneous equations (system of equations) in two variables | 199 |
| 12. Solving problems with simultaneous equations (systems of equations) | 204 |
| 13. Linear systems of equations in more than two unknowns (higher level courseware) | 209 |
| 14. Practical exercises | 213 |
| Congruent transformations | 216 |
| Congruent transformations 1. The concept of geometric transformation, examples of geometric transformations | 216 |
| 2. Line reflection (reflection about a straight line) in the plane | 218 |
| 3. Axially symmetric figures | 221 |
| 4. Point reflection in the plane | 225 |
| 5. Centrally symmetric figures | 228 |
| 6. Applications of point reflection | 231 |
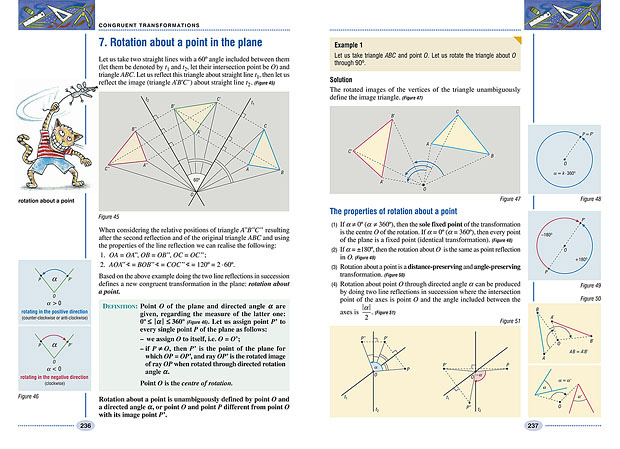
| 7. Rotation about a point in the plane | 236 |
| 8. Applications of rotation about a point I . | 239 |
| 9. Applications of rotation about a point II | 244 |
| 10. Parallel translation. Vectors | 246 |
| 11. Operations with vectors | 251 |
| 12. Congruence of figures | 256 |
| Statistics | 260 |
| 1. The representation of data | 260 |
| 2. The description of data | 264 |
A kiadvány bevezetője
| Guide to use the course book. The notations and highlights used in the book help with acquiring the courseware. - The train of thought of the worked examples show samples how to understand the methods and processes and how to solve the subsequent exercises. - The most important definitions and theorems are denoted by colourful highlights. - The parts of the courseware in small print and the worked examples noted in claret colour help with deeper understanding of the courseware. These pieces of knowledge are necessary for the higher level of graduation. - Figures, the key points of the given lesson, review and explanatory parts along with interesting facts of the history of mathematics can be found on the margin. The difficulty level of the examples and the appointed exercises is denoted by three different colours: Yellow: drilling exercises with basic level difficulty; the solution and drilling of these exercises is essential for the progress. Blue: exercises the difficulty of which corresponds to the intermediate level of graduation. Claret: problems and exercises that help with preparing for the higher level of graduation. These colour codes correspond to the notations used in the Colourful mathematics workbooks of Mozaik Education. The workbook series contains more than 3000 exercises which are suitable for drilling, working on in lessons and which help with preparing for the graduation. The end results of the appointed exercises can be found on the following website: www.mozaik.info.hu. Website www.mozaweb.hu offers more help material for processing with the course book. |
