Angol nyelvű matematika

|
10. évfolyam, 3. kiadás (2021. 11. 03.) Mozaik Kiadó
méret: 170x240 mm
terjedelem: 256 oldal
tanterv: NAT 2012
6 780 Ft
Kosárba  |
| A kiadvány az MS-2310 Sokszínű matematika 10. c. kötet angol nyelvű változata. This book is the English version of the former Hungarian market leader textbook titled Sokszínű matematika 10. |
Kapcsolódó kiadványok
| A kiadvány digitális változata a könyvben levő kóddal ingyenesen elérhető * A kiadvány hátsó borítójának belső oldalán található egyedi kóddal a kiadvány digitálisan is elérhető. Az aktivált kódokkal 1 éves DÍJMENTES hozzáférést ajándékozunk a kiadvány digitális változatához. Az aktiválás a www.mozaweb.hu/aktivalas oldalon, a Fiókom/Új kód aktiválása menüpontban érhető el. |
Mintaoldalak
Tartalomjegyzék
| Mathematical reasoning | 10 |
| 1. What does it imply? | 10 |
| 2. The pigeonhole principle | 21 |
| 3. Arrangement (ordering) problems | 29 |
| 4. Picking problems | 32 |
| Computing the root | 36 |
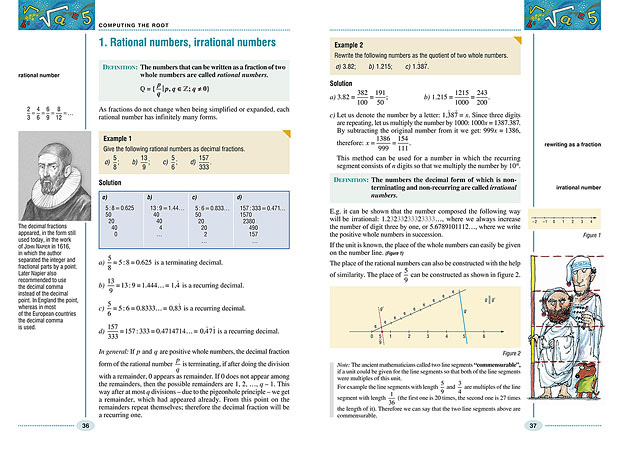
| 1. Rational numbers, irrational numbers | 36 |
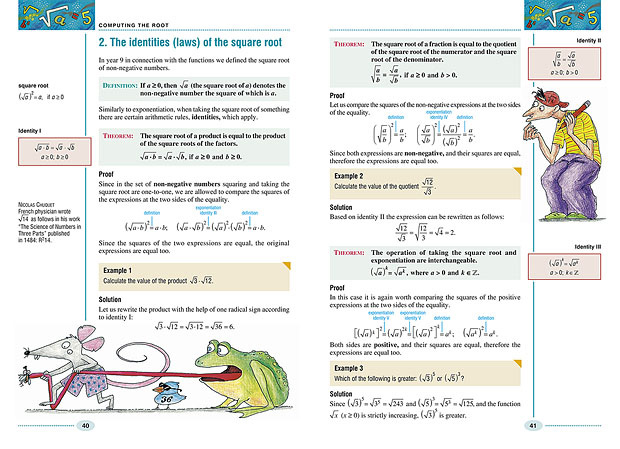
| 2. The identities (laws) of the square root | 40 |
| 3. Applying the identities (laws) of the square root | 44 |
| 4. The nth root of numbers | 50 |
| 5. The identities (laws) of the nth root . | 53 |
| The quadratic equation | 60 |
| 1. The quadratic equation and function | 60 |
| 2. The quadratic formula | 64 |
| 3. The zero product form. The relation between the roots and the coefficients | 69 |
| 4. Equations of higher degree which can be reduced to quadratic equations | 74 |
| 5. Quadratic inequalities | 80 |
| 6. Parametric quadratic equations (higher level courseware) | 84 |
| 7. Equations involving square roots | 90 |
| 8. Quadratic simultaneous equations | 96 |
| 9. Arithmetic and geometric mean | 101 |
| 10. Extreme value exercises (higher level courseware) | 106 |
| 11. Problems leading to quadratic equations | 110 |
| Geometry | 116 |
| Widening the knowledge about circles | 116 |
| 1. Reminder | 116 |
| 2. The theorem of the central and inscribed/tangent-chord angles | 117 |
| 3. The theorem of inscribed angles; the arc of viewing angles | 121 |
| 4. The theorem of inscribed quadrilaterals (higher level courseware) | 125 |
| The similarity transformation and its applications | 129 |
| 1. Parallel intercepting lines, parallel intercepting line segments (higher level courseware) | 129 |
| 2. The angle bisector theorem (higher level courseware) | 135 |
| 3. The transformation of central dilation (or homothety) | 137 |
| 4. The similarity transformation | 141 |
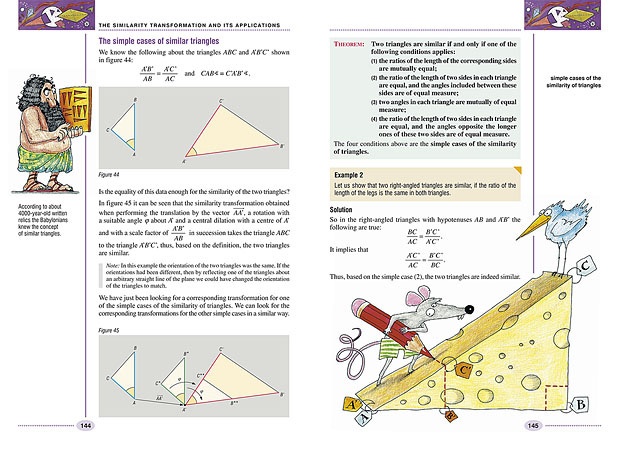
| 5. Similarity of figures; the simple cases of similar triangles | 143 |
| 6. A few applications of similarity | 147 |
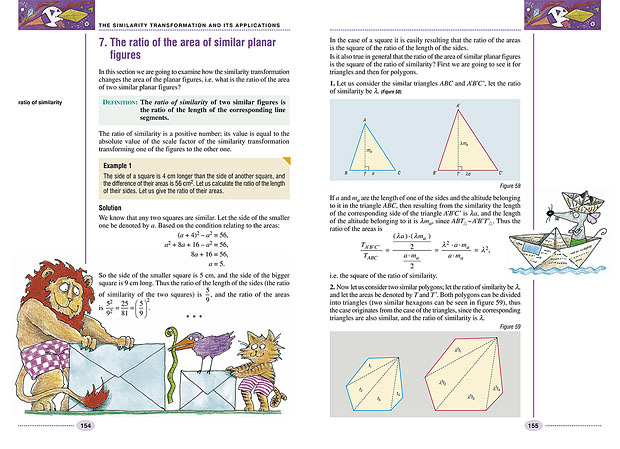
| 7. The ratio of the area of similar planar figures | 154 |
| 8. The ratio of the volume of similar solids | 158 |
| Trigonometric functions of acute angles | 161 |
| 1. Determining distances with the help of similarity | 161 |
| 2. Trigonometric functions of acute angles | 164 |
| 3. Relations between the trigonometric functions of acute angles | 168 |
| 4. Trigonometric functions of special angles | 172 |
| 5. Determining several data of a triangle with the help of trigonometric functions | 175 |
| 6. Calculations in the plane and in space with the help of trigonometric functions | 180 |
| Vectors | 184 |
| 1. The concept of a vector; the sum and the difference of vectors; scalar multiplication of vectors (reminder) | 184 |
| 2. Expressing vectors as the sum of components in different directions | 188 |
| 3. Applying vectors in the plane and in space | 194 |
| 4. Vectors in the coordinate system, the coordinates of a vector, operations with vectors given with coordinates | 199 |
| Trigonometric functions | 204 |
| 1. The definition and the simple properties of the sine and the cosine function | 204 |
| 2. The graph of the sine function | 209 |
| 3. The graph of the cosine function, equations, inequalities | 214 |
| 4. The tangent and the cotangent function | 221 |
| 5. Compound exercises and applications . | 228 |
| 6. Geometric applications | 232 |
| Calculation of probability | 238 |
| 1. Events | 238 |
| 2. Operations with events | 243 |
| 3. Experiments, frequency, relative frequency, probability | 248 |
| 4. The classical model of probability .. | 251 |
A kiadvány bevezetője
| Guide to use the course book. The notations and highlights used in the book help with acquiring the courseware. - The train of thought of the worked examples show samples how to understand the methods and processes and how to solve the subsequent exercises. - The most important definitions and theorems are denoted by colourful highlights. - The parts of the courseware in small print and the worked examples noted in claret colour help with deeper understanding of the courseware. These pieces of knowledge are necessary for the higher level of graduation. - Figures, the key points of the given lesson, review and explanatory parts along with interesting facts of the history of mathematics can be found on the margin. The difficulty level of the examples and the appointed exercises is denoted by three different colours: Yellow: drilling exercises with basic level difficulty; the solution and drilling of these exercises is essential for the progress. Blue: exercises the difficulty of which corresponds to the intermediate level of graduation. Claret: problems and exercises that help with preparing for the higher level of graduation. These colour codes correspond to the notations used in the Colourful mathematics workbooks of Mozaik Education. The workbook series contains more than 3000 exercises which are suitable for drilling, working on in lessons and which help with preparing for the graduation. The end results of the appointed exercises can be found on the following website: www.mozaik.info.hu. Website www.mozaweb.hu offers more help material for processing with the course book. |








